Interdisciplinary Note (6 of 16)
Many students have difficulty with topic of magnetism because, unlike the electric field, the magnetic field seems to be positioned so strangely in space which makes it difficult to get conceptually oriented. The purpose of the following discussion is to give you a sense of how magnetism looks in the light of special relativity. Special relativity is not an MCAT topic. The whole purpose here is to make magnetism easier to adjust to conceptually. Thinking on these concepts a bit will help improve your intuition about electromagnetism in general.
Fundamental to the topic Electromagnetic Induction are Maxwell's equations (do not fear these! they are not for memorization or direct problem solving at this level!) These equations are among the most important in physical science, describing how a changing electric field induces a magnetic field, and how a changing magnetic field induces an electric field, and predicting the propagation of light as electromagnetic phenomena. Through special relativity, Einstein reinterpreted electromagnetic phenomena to show that the electric force and the magnetic force were relativistic flip sides of the same underlying electromagnetic force. He readjusted our perspective on Maxwell's equations, saying that they imply 'an asymmetry not inherent in the phenomena' not that they are wrong but that their form of expression leads to a misleading asymmetry between the magnetic field and the electric field arising from a resting observer's terminology.
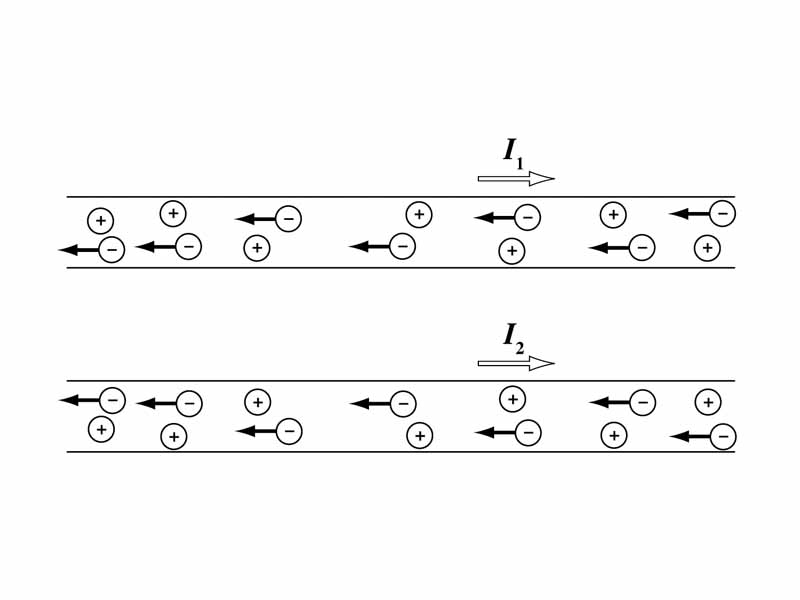
Okay. The example of the magnetic force between two current carrying wires is helpful to show how this works. I think this example can help quite a bit. From a resting perspective, two parallel wires carrying current in the same direction attract one another by magnetic force. Everybody learns this. There is no electric field around the wires because the wires are electrically neutral overall (there are the same number of positive and negative charges in a given wire segment, though one type of charge is moving). From our perspective, the overall charge density is neutral, although there is a current. Being electrically neutral, there is no electric field around the wire, no electric force, but still they are attracted. Early researchers called this force the magnetic force, distinguishing it from the electrostatic force given by Coulomb's Law.
Let's apply special relativity to approach the problem conceptually. Imagine we are moving along with the charges on one of the wires. What we say is that we are now in the relativistic frame of those moving charges. Looking over at the other wire, what do we see? We see like charges which appear stationary (they are moving along with us) and unlike charges moving in the opposite direction. Everybody who ever watched PBS science specials as a kid has heard how special relativity predicts the contraction of space at high velocity. This is called Lorentz contraction. Due to Lorenz contraction, the space of the unlike charges moving relative to us on the other wire is compressed. This means that the electric field density of these charges appears greater in this relativistic frame. With space contracted, the electric field of the opposite charges from the other wire is more dense from our moving perspective. So while in our original 'resting frame' there is no net electric field, in the frame of the moving charges on one of the wires, there does appear to be a net electric field generated by the unlike charges on the other wire, which causes the wires to attract one another.
What Einstein is saying is that the 'asymmetry' between the electric and magnetic field is just a convention. The difference between the electric and magnetic fields is not inherent to the phenomena. The asymmetry occurs to us as resting observers because of our point of view. The mathematical way to say this is that the electric and magnetic fields are not isolated vectors. They transform into each other when you change frames. Really, they fit into a different object, a 'tensor', that behaves very nicely under Einstein's relativity. Maxwell thought he had two vector fields, electric charges, and four dynamic equations connecting them. Einstein realized that there was one electromagnetic field tensor.
Now, of course, the MCAT will never ask you about the relativistic basis of the apparent asymmetry of electricity and magnetism. Nevertheless, I have found that students feel more comfortable within basic magnetism through this understanding. Now you can see what all the right hand rules are doing, what the purpose is of drawing the magnetic field looping around a current. It is just our way of orienting ourselves in space to account for the directionality of Lorenz contraction to predict whether or not, and in which direction, magnetic force is occurring.