Entropy of mixture.

Statistical mechanics has its quantitative side, which is beyond the scope of the MCAT, and its conceptual side, which provides some helpful perspective for understanding fundamental thermodynamics ideas.

Statistical mechanics approaches particle motion within a thermodynamic system as a distribution of states. Some particles move slowly. Some move fast. Most are in between. The characteristic curve describing the distribution of molecular speed in a sample of gas is called the Maxwell/Boltzman distribution.

One thing about the Maxwell/Boltzman distribution is that he higher the temperature, the wider the distribution. What this means is that there are more possible combinations of motion available to a thermodynamic system at a higher temperature. In other words, entropy increases with higher temperature.

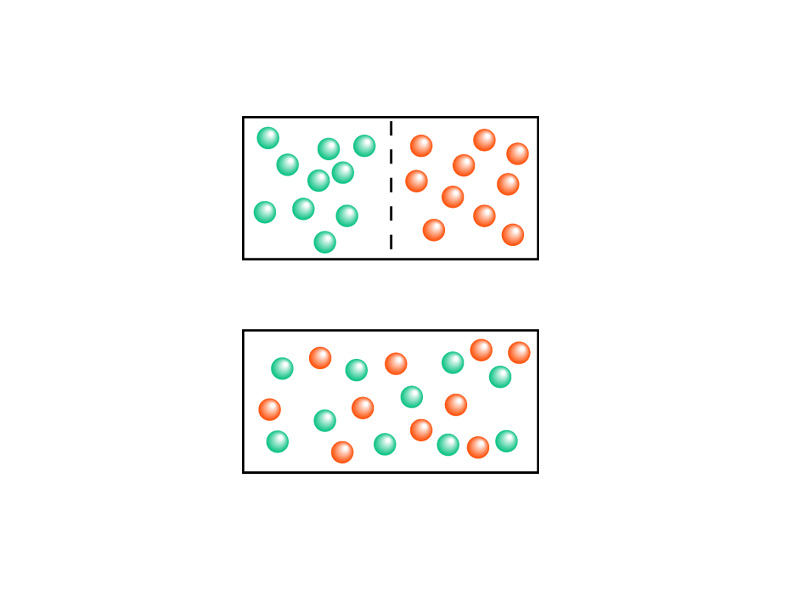
The more possible states available to the molecules, the greater the total chaos. The greater the number of microstates in the ensemble underlying the macrostate. Such a macrostate is more likely because there are so many combinations that produce that macrostate. Picture all of the molecules in a container shifting through all possible configurations. When will it hit the one where all the molecules are on one side of the container and the other side is empty? With Avogadro's number of particles, it could play through possible combinations and not hit that one microstate. The gas does not diffuse backwards. The heat does not flow backwards from the cold body back into the hot body.

In these terms, the second law is telling us that molecules will not become more restricted in possibilities, without there being a balancing increase in disorder to the surroundings. It is much more likely for a deck of cards sorted into suits to fall to the floor and spontaneously become chaotic than for a deck which is mixed to fall to the floor and spontaneously sort into suits. One state, the higher entropy, is much more probable than the other. You could sort the cards yourself and impose order on the system from without.

Events occur irreversibly because the direction is going towards greater statistical likelihood. If the future were as likely as the past, events could go backwards as well as forwards.